Research Posters 11, p. 53
Comparative Evaluation of New and Old ProTaper F3 Instruments using
Physico-mathematical Analysis
Evgeny Rzhanov (Department of Operative Dentistry and Endodontics, Moscow State University of Medicine and Dentistry, Moscow, Russia)
Tatiana Belyaeva (Department of Operative Dentistry and Endodontics, Moscow State University of Medicine and Dentistry, Moscow, Russia)
Aim
To evaluate and compare basic properties of new and old ProTaper F3 instruments by using physico mathematical analysis of its design features.
Methodology
20 ProTaper F3 instruments (Dentsply Maillefer, Switzerland) were included in this study: 10 of the new design and 10 of the old design. A scanning electron photomicrographs were taken of each instrument Then all instruments were sectioned transversely at 1. 3, 6 and 10 mm from the tip and also examined by SEM. All images were captured digitally and the following design parameters were assessed by a direct measurement technique: helical angle, pitch length, depth of flutes, external and internal diameters, angles of cutting blades. All measurement values were analyzed mathematically to evaluate and compare instrument properties such as: flexibility, self-feeding force component, volume of fluting along the axis, safe depth of cutting per unit step of treatment.
Results
New F3 instrument showed significantly less flexibility than the old F3 instrument at 1 mm and 3 mm from the tip (p<0.05). Where was no statistically significant difference in flexibility between new F3 and the old F3 at the 6 mm from the tip (p>0.05). At the 10 mm from the tip new F3 showed greater flexibility than the old F3 (p<0.05). New F3 has less self-feeding effect than the old F3. New F3 has less volume of fluting (p<0.05) and less safe depth of cutting per unit step of treatment than the old F3 (1,4 mm and 1,6 mm respectively).
Conclusions
New ProTaper F3 differs from the old ProTaper F3 in design that determines its different properties."
Tatiana Belyaeva (Department of Operative Dentistry and Endodontics, Moscow State University of Medicine and Dentistry, Moscow, Russia)
Aim
To evaluate and compare basic properties of new and old ProTaper F3 instruments by using physico mathematical analysis of its design features.
Methodology
20 ProTaper F3 instruments (Dentsply Maillefer, Switzerland) were included in this study: 10 of the new design and 10 of the old design. A scanning electron photomicrographs were taken of each instrument Then all instruments were sectioned transversely at 1. 3, 6 and 10 mm from the tip and also examined by SEM. All images were captured digitally and the following design parameters were assessed by a direct measurement technique: helical angle, pitch length, depth of flutes, external and internal diameters, angles of cutting blades. All measurement values were analyzed mathematically to evaluate and compare instrument properties such as: flexibility, self-feeding force component, volume of fluting along the axis, safe depth of cutting per unit step of treatment.
Results
New F3 instrument showed significantly less flexibility than the old F3 instrument at 1 mm and 3 mm from the tip (p<0.05). Where was no statistically significant difference in flexibility between new F3 and the old F3 at the 6 mm from the tip (p>0.05). At the 10 mm from the tip new F3 showed greater flexibility than the old F3 (p<0.05). New F3 has less self-feeding effect than the old F3. New F3 has less volume of fluting (p<0.05) and less safe depth of cutting per unit step of treatment than the old F3 (1,4 mm and 1,6 mm respectively).
Conclusions
New ProTaper F3 differs from the old ProTaper F3 in design that determines its different properties."
Introduction
Over the last twenty years nickel-titanium rotary instruments technology grows rapidly. Every year several new nickeltitanium rotary systems enter the market. While some of them are usefully employed by the dentists worldwide, the others become a history.
The Pro Taper system appears on the market at the beginning of the 2000's and now it became the best-selling rotary nickel-titanium system in the world. Throughout its existence the Pro Taper system was modified and extended more than once. The last modifications affected the design of the Pro Taper F3 instrument.
In our view it appears to be interesting to carry out a comparative analysis of the new and old versions of the Pro Taper F3 instruments to evaluate the difference between it and to understand how these changes can improve the instrument properties. There are few essential properties determining the operation peculiarities of the instrument: flexibility, cutting efficiency and self-feeding (screwing) effect.
The Pro Taper system appears on the market at the beginning of the 2000's and now it became the best-selling rotary nickel-titanium system in the world. Throughout its existence the Pro Taper system was modified and extended more than once. The last modifications affected the design of the Pro Taper F3 instrument.
In our view it appears to be interesting to carry out a comparative analysis of the new and old versions of the Pro Taper F3 instruments to evaluate the difference between it and to understand how these changes can improve the instrument properties. There are few essential properties determining the operation peculiarities of the instrument: flexibility, cutting efficiency and self-feeding (screwing) effect.
Aim
To evaluate and compare the basic properties of new and old Pro Taper F3 instruments by using physics-mathematics analysis of its design features.
Methodology
Twenty Pro Taper F3 instruments (Dentsply Maillefer, Switzerland) were included in this study: 10 of the new design and 10 of the old design. A scanning electron photomicrographs of each instrument were taken. Then all instruments were mounted in a transparent tubular mold and sectioned transversely at 1, 3, 6 and 10 mm from the tip. All cross sections were examined by SEM; images were captured digitally and the following design parameters were assessed by a direct measurement technique: rake angles of cutting blades, internal diameters, helical angles, pitch length, depth of flutes according to Fig 1.
All measurement values were analyzed mathematically to compare the following instruments properties: cutting ability, flexibility, self-feeding effect, volume of fluting along the axis, safe depth of cutting per unit step of treatment.
All measurement values were analyzed mathematically to compare the following instruments properties: cutting ability, flexibility, self-feeding effect, volume of fluting along the axis, safe depth of cutting per unit step of treatment.

Fig 1
Basic design parameters of the Pro Taper F3 instrument. α — clearance angle. β — wedge angle. γ — rake angle.
Dint — internal diameter. Dext — external diameter.
Basic design parameters of the Pro Taper F3 instrument. α — clearance angle. β — wedge angle. γ — rake angle.
Dint — internal diameter. Dext — external diameter.
Cutting ability
From the physical standpoint cutting ability is determined by the forces applied to the instrument. The smaller cutting force applied at the same rotational speed the higher cutting ability of the instrument.
During cutting process there is a main force, acting on the face surface of the instrument blade — the force of normal pressure from the cut dentine chip — N.
The vector component of normal pressure force — the friction force F — acts along the cut dentine surface in the direction opposite to the vector of linear speed V:
During cutting process there is a main force, acting on the face surface of the instrument blade — the force of normal pressure from the cut dentine chip — N.
The vector component of normal pressure force — the friction force F — acts along the cut dentine surface in the direction opposite to the vector of linear speed V:
where the angle yis the negative rake angle of endodontic instrument
The second vector component of normal pressure force is the force R acting in the direction opposite to normal n to the cut dentine surface:
The second vector component of normal pressure force is the force R acting in the direction opposite to normal n to the cut dentine surface:
When the flank surface of instrument blade touches the cut dentine surface another force Fα arises. This force arises in the case of a very small clearance angle when the rebound of elastically deformed dentine exceed the clearance space. Fα directs perpendicularly to the flank surface, prevent the instrument to go deeper into dentine and also could be represented through x and y projections.
General equation for cutting process could be written as the sum of vectors:
General equation for cutting process could be written as the sum of vectors:
Cutting ability of the instrument depends on the sum of these vectors and consequently on the angles of cutting blade.
Flexibility
The free energy of the unit volume of the elastically bended rod has been calculated in theory of elasticity as:
where E — the elastic modulus, x — the displacement of the cross section under the bending and R — curvature radius. The integral of ( 1 ) over the cross section of the rod gives us the free energy of unit length of the bended rod:
where df — the element of surface of the cross section. The bending inertia of cross section could be introduced as:
Then the free energy of the unit length of the rod has the form:
The curvature of bending 1/R is proportional to acting momentum of forces for given cross section of rod:
For the round cross-section with radius rint the center of inertia is located at the center of circle and the value of Iy is:
(9)
We determine the flexibility as the ability to bend with curvature 1/R under the action of momentum My . So it is reasonable to measure the flexibility Fas the reverse value of free energy of unit length of bended rod:

(10)
As a result, flexibility depends on modulus E of material and the internal radius of cross section of degree four.
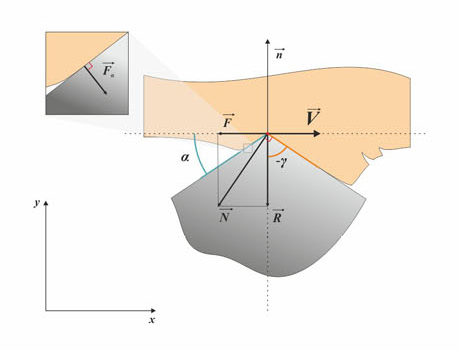
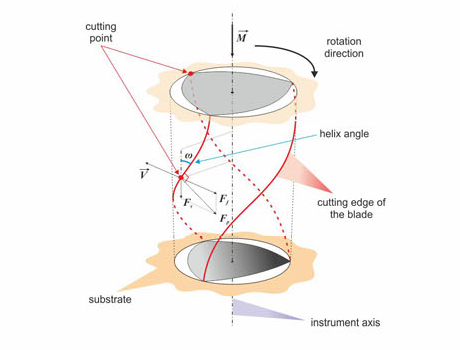
Fig 2
System of forces ent acting during on cutting the blade process of nickel-titanium (explanation is rotary in the instrumtext).
System of forces ent acting during on cutting the blade process of nickel-titanium (explanation is rotary in the instrumtext).
Fig 3
Interaction pattern full-rotation between instrument blade and root canal wall during full-rotation preparation (explanation and root is in the text).
Interaction pattern full-rotation between instrument blade and root canal wall during full-rotation preparation (explanation and root is in the text).
Self feeding effect
During root canal preparation all fluted instruments (if the fluting has the same direction with the rotation motion) are drawn into the canal due to the rotation even in the absence of vertical pressure. In general engineering this effect is called the self feeding effect.
Figure 3 schematically displays the instrument fragment with three blades (plotted with red lines). The torque M applied to the instrument provides its rotational movement. The cutting points belonging to cutting edges move with linear speeds V which is in direct relationship to the radius of the instrument. Each point belongs to the elementary area located nearby the cutting edge and subjected to the force of normal pressure from the cut substrate. This force in Figure 3 denoted as Fp and directed perpendicular to the elementary area. The normal pressure force Fp could be split into mutually perpendicular components: Ft which is directed against the speed vector V and is named frictional force; and Fs directed towards the apical part of the canal and is named self feeding force. Thus even in the absence of vertical force from the operator the instrument is drawn into the canal by force resulting from rotation. The self feeding force rises with the increase of helical angle w under otherwise equal conditions.
Figure 3 schematically displays the instrument fragment with three blades (plotted with red lines). The torque M applied to the instrument provides its rotational movement. The cutting points belonging to cutting edges move with linear speeds V which is in direct relationship to the radius of the instrument. Each point belongs to the elementary area located nearby the cutting edge and subjected to the force of normal pressure from the cut substrate. This force in Figure 3 denoted as Fp and directed perpendicular to the elementary area. The normal pressure force Fp could be split into mutually perpendicular components: Ft which is directed against the speed vector V and is named frictional force; and Fs directed towards the apical part of the canal and is named self feeding force. Thus even in the absence of vertical force from the operator the instrument is drawn into the canal by force resulting from rotation. The self feeding force rises with the increase of helical angle w under otherwise equal conditions.
Fig 4


Fig 5
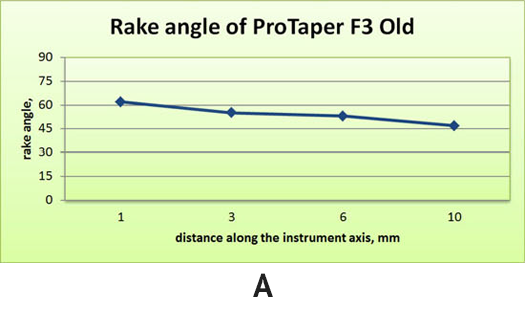
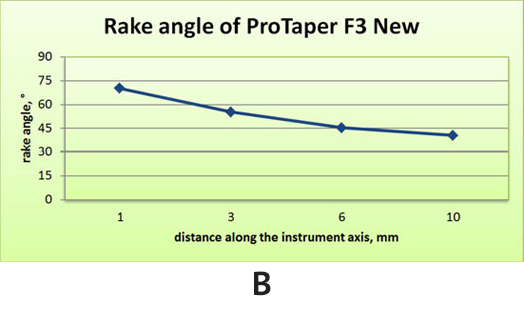
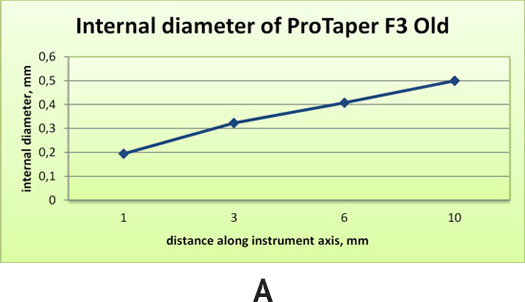
Fig 6
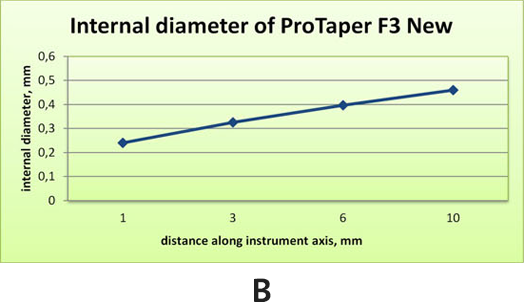
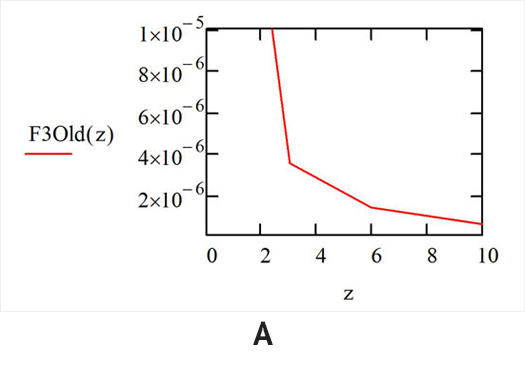
Fig 7
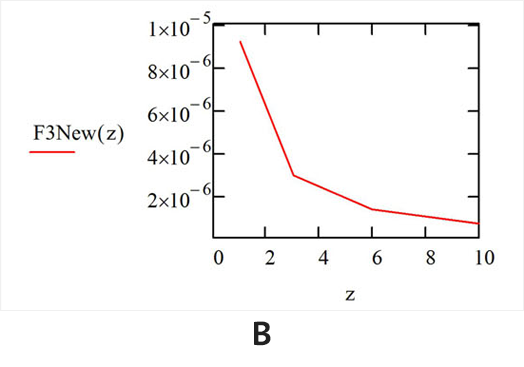

Fig 8
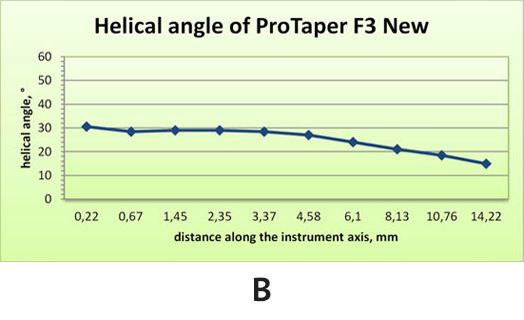
Fig 9

Fig 10
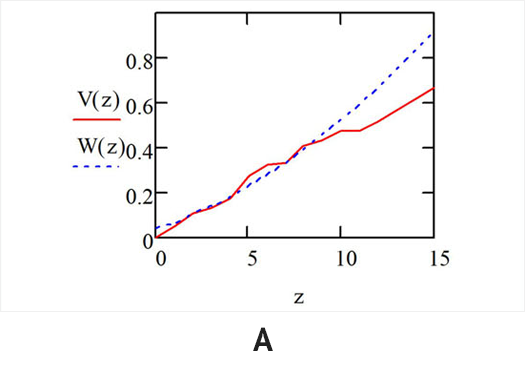
Fig 11
Volume of fluting and
Safe operating depth per unit step of treatment
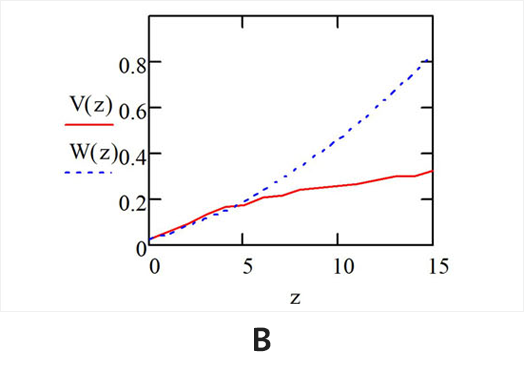
The important condition of instrument safety is the accordance of flute volume and the volume of cut dentine during one step of treatment. The volume of cut dentine should be less than flute volume of the working part of the instrument acting on each stage of operation. The flute volume (red line on Fig. 11) was observed by measuring parameters of instrument cross sections at z=1mm, 3mm, 6mm and 10mm. These parameters are: external radius rext, internal radius rint, and the form of helical line of flute.
The volume of cut dentine has been calculate taking into account instrument geometry, its taper k at the tip and the permissible depth of penetration into the canal for the given step of treatment, which might be also termed "safe operating depth" (SOD). The formula for the volume of cut dentine is:
The volume of cut dentine has been calculate taking into account instrument geometry, its taper k at the tip and the permissible depth of penetration into the canal for the given step of treatment, which might be also termed "safe operating depth" (SOD). The formula for the volume of cut dentine is:
(12)

(12)
This function is the blue line on Fig.11. Here t designates SOD, r0 — the radius of the tip, and k — the taper at the thinnest part near to tip.
In order to find the SOD t using MathCAD it needs firstly to plot the experimental data for the volume of flute V(z) and then to plot the W(z) using instrument data: r0 and k at the small value of SOD t. Third step is variation of parameter k to make W(z) parallel to volume of flute V(z) at the value of z in the range 1-5mm (at the tip).
Last step is the increase of the SOD t to make it coincide with plot of flute volume at small values of z.
So we can determine the safe operating depth per unit step of root canal preparation. After each step of preparation instrument must be cleaned out.
In order to find the SOD t using MathCAD it needs firstly to plot the experimental data for the volume of flute V(z) and then to plot the W(z) using instrument data: r0 and k at the small value of SOD t. Third step is variation of parameter k to make W(z) parallel to volume of flute V(z) at the value of z in the range 1-5mm (at the tip).
Last step is the increase of the SOD t to make it coincide with plot of flute volume at small values of z.
So we can determine the safe operating depth per unit step of root canal preparation. After each step of preparation instrument must be cleaned out.
Results
Cutting ability. There is no statistically significant difference between rake angle values of Old and New Pro Taper F3 instruments (p>.05) (Fig. 9). So the cutting ability of both instrument types is nearly identical.
Flexibility. New F3 instrument showed significantly less flexibility than the old F3 instrument at 1 mm and 3mm from the tip (p<.05) (Fig.10). Where was no statistically significant difference in flexibility between new F3 and the old F3 at the 6mm from the tip (p > .05).Atthe 10mm from the tip new F3 showed greater flexibility than the old F3 (p<.05).
Self-feeding effect. New Pro Taper F3 instrument has less helical angle along the entire length except first millimeter (Fig.5). So the new F3 has less self-feeding effect than the old F3.
Volume of fluting and safe operating depth. New F3 has significantly less volume of fluting than the Old F3: 3.31 mm3 and 5.55mm3 respectively (p < .05) and less safe depth of cutting per unit step of treatment: 1,4mm and 1,6mm respectively (Fig.11).
Flexibility. New F3 instrument showed significantly less flexibility than the old F3 instrument at 1 mm and 3mm from the tip (p<.05) (Fig.10). Where was no statistically significant difference in flexibility between new F3 and the old F3 at the 6mm from the tip (p > .05).Atthe 10mm from the tip new F3 showed greater flexibility than the old F3 (p<.05).
Self-feeding effect. New Pro Taper F3 instrument has less helical angle along the entire length except first millimeter (Fig.5). So the new F3 has less self-feeding effect than the old F3.
Volume of fluting and safe operating depth. New F3 has significantly less volume of fluting than the Old F3: 3.31 mm3 and 5.55mm3 respectively (p < .05) and less safe depth of cutting per unit step of treatment: 1,4mm and 1,6mm respectively (Fig.11).
Conclusions
Pro Taper F3 New differs from the Pro Taper F3 Old in design that determines its different properties. Pro Taper F3 Old by the physical measurements is better than Pro Taper F3 New instrument: the flexibility is better and the flute volume is higher too.
